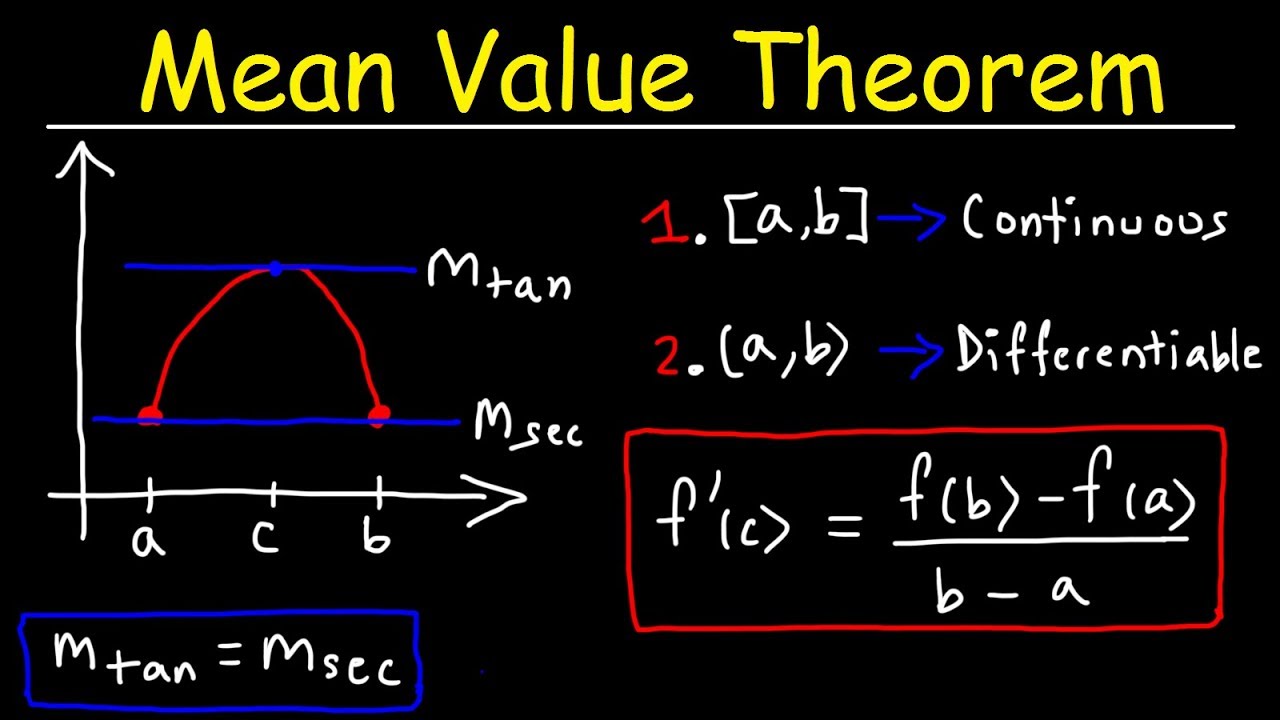
Mean value theorem is one of the important theorems used in both differential and integral calculus. It helps us to understand the identical behavior of different functions and has very important consequences in differential calculus.  This theorem defines the derivative of a differential and continuous function. The hypothesis and conclusion of this theorem show some similarities to those of the Intermediate value theorem.

The mean value theorem is abbreviated as MVT. This theorem states that let f(x) is a function which is continuous in [a,b] and differentiable in (a,b), then there exists a real number c, such that c belongs to (a,b) and  f ‘(c) =  (f(b) – f(a)) /(b – a). 

Physical Interpretation of Mean Value Theorem

Since (f(b)−f(c))/(b−a) is the average change in the function over [a, b], and f'(c) is the instantaneous change at ‘c’, MVT states that at some interior point the instantaneous change is equal to the average change of the function over the interval.

Important results

The following are some important results used in MVT.

1. Let f(x) and g(x) be functions such that, f and g are continuous in interval [a,b] and differentiable on interval (a,b),

f'(x) = g'(x), x ∈ (a,b), then f(x) – g(x) is constant in [a,b]

2. Let the function be f such that it is continuous in interval [a,b] and differentiable on interval (a,b), then f'(x) = 0, x ∈ (a,b), then f(x) is constant in [a,b].
3. Strictly Decreasing Function

Let the function be f such that, continuous in interval [a,b] and differentiable in interval (a, b)

f'(x) < 0, x ∈ (a,b), then f(x) is a strictly decreasing function in [a,b].

4. Strictly Increasing Function

Let the function be f such that, continuous in interval [a, b] and differentiable in interval(a,b)

f'(x) > 0, x ∈ (a,b), then f(x) is strictly an increasing function in [a,b].

MVT for Derivatives

We approximate the derivative of any function using the MVT. The theorem can build a relationship between the secant line on a curve and the slope of a tangent line. If f is differentiable over (a,b) and continuous over [a,b] then there exists a point

c in such a way that f′(c) = {f(b)−f(a)}/(b−a).

This shows that the actual slope is equal to the average slope at some point in the closed interval. Geometrically, we can say that between two endpoints of the curve, we have at least one point on the curve where the slope of the tangent line is equal to the slope of the secant line passing through A and B.

Derivative 

The derivative of a function is represented by f’(x). The derivative is a value that changes with respect to its input. Differentiation is the method of finding the derivative of a function. A function that shows the rate of change of the other function can be called the derivative of that function. 

It is represented as a dependent variable in terms of an independent variable through an equation. The change in the slope of a function is known as the derivative. The slope is denoted as follows:

Slope = dy/dx

Let us consider a function that involves the change in velocity of a car moving from one point to another. The change in velocity is dependent on the speed and direction in which the car is moving. To find the acceleration, we need the limits of the function. The theory of derivative is derived from limits. 

Some Derivative rules

1. Constant rule : (d/dx)C = 0
2. Power rule: (d/dx) xn = nxn-1
3. Constant multiple rule: (d/dx) [c.u] = c. du/dx
4. Product rule: (d/dx) (u.v) = u. (dv/dx) + v. (du/dx)
5. Quotient rule: (d/dx) (u/v) = [v.(du/dx) – u.(dv/dx)]/v2

Few derivative examples are given below. 

1. Find the derivative of x4+2x+7

Solution:

By the power rule, (d/dx)x4 = 4x3

(d/dx)2x = 2

By constant rule, (d/dx)7 = 0

Hence the derivative of x4+2x+7 = 4x3 +2

 

2. Find the rate of change of the area of a circle w.r.t the radius. How fast is the area changing with respect to radius when the radius is 4 cm?

Solution:

Area of circle, A = πr2 

dA/dr = 2πr

rate of change of area of a circle w.r.t the radius = 2πr

When r = 4 cm, dA/dr = 2π(4) = 8π cm.